Strong Bounds for 3-Progressions | Breakthroughs

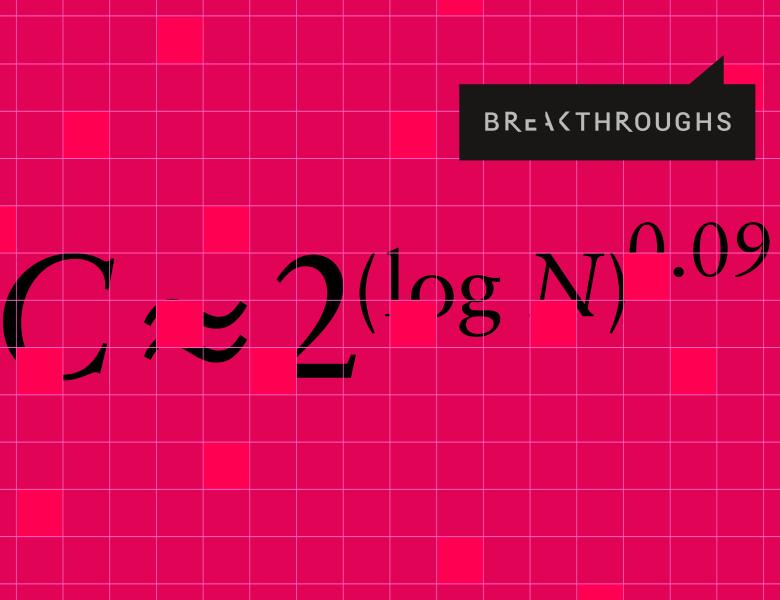
Abstract: Suppose you have a set $S$ of integers from $\{1,2,…,N\}$ that contains at least $N / C$ elements. Then for large enough $N$, must $S$ contain three equally spaced numbers (i.e., a 3-term arithmetic progression)?

In 1953, Roth showed that this is indeed the case when $C \approx (\log \log N)$, while Behrend in 1946 showed that $C$ can be at most $2^{\Omega(\sqrt{\log N})}$. Since then, the problem has been a cornerstone of the area of additive combinatorics. Following a series of remarkable results, a celebrated paper from 2020 due to Bloom and Sisask improved the lower bound on $C$ to $C = (\log N)^{(1+c)}$, for some constant $c > 0$.

This talk will describe a new work showing that the same holds when $C \approx 2^{(\log N)^{0.09}}$, thus getting closer to Behrend’s construction.

Based on joint work with Zander Kelley.